When we determine the force constant of the spring, we are only finding out about the stiffness of that particular spring. Also, we can compare the stiffness of different materials e.g. copper is stiffer than lead.
In this article we would be looking at What is Force Constant and Units.
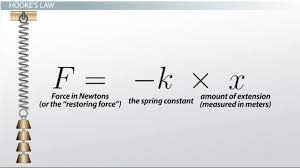
Force constant is sometimes called spring constant or stiffness or elastic constant. So, it is defined as the force per unit extension. The force constant k of the spring is given by the equation
K = F/X where F is the force applied and X is the extension.
From the graph above, the graph has the force on the vertical axis and the extension on the horizontal axis. This is a departure from the conventional way of plotting the result because the gradient of the straight section of this graph turns to be an important quantity, known as the force constant of the spring.
The extension x is directly proportional to the applied force F. The behaviour of the spring in the linear region OA of the graph can be expressed by the following equation. Force constant = the slope (gradient) of the graph.
X directly proportional to F
F = Kx
The k is the proportionality constant which is referred to in physics as the force constant.
A stiffer spring will have a larger value for k. Beyond point A, the graph is no longer a straight line; its gradient changes and can no longer use the equation F = Kx.
Unit And Dimension Of Force Constant
Recall, K = F/X
F is the force with the Unit Newton (N)
X is the extension with a unit of meter (m)
Therefore, the unit of force constant is Newton per meter (Nm-1)
To get the dimension of force constant, we need to know the dimension of force and extension
The dimension of force is MLT-2 and that of extension is L
Therefore, the dimension of force constant is MLT-2/L, which then is [MT-2]
I hope you find this article helpful as well as interesting.
